Problem in this Issue (Vol 411/09/2011)
FOR LOWER SECONDARY SCHOOLS
T1/411 (For 6 grade). The natural numbers  are arranged in some order in a
are arranged in some order in a  square table, each square contians one number. Prove that there exits two adjacent square (that is two square having a common edge or common vertex) such that the difference between the corresponding assigned numbers is not smaller than 2012.
square table, each square contians one number. Prove that there exits two adjacent square (that is two square having a common edge or common vertex) such that the difference between the corresponding assigned numbers is not smaller than 2012.
T2/411 (For 7 grade). Find the value of the following 2009-terms sumT4/411.  is a point in the interior of a triangle
is a point in the interior of a triangle  . Let
. Let  be respectively the centroid of triangles
be respectively the centroid of triangles 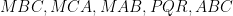 . Prove that points
. Prove that points 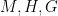 are colinear.
are colinear.
T5/411. Let FOR UPPER SECONDARY SCHOOLS
T6/411. The incircle  of a triangle
of a triangle  touches
touches 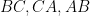 at
at 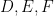 respectively. The line passing through
respectively. The line passing through 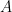 and parallel to
and parallel to  meets
meets  at
at  is the midpoint of
is the midpoint of  . Prove that
. Prove that 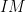 is perpendicular to
is perpendicular to 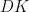 .
.
T7/411. Slove the systerm of the equations
T8/411. Let  be real numbers such that the equation
be real numbers such that the equation  has two solutions, both are in the closed interval
has two solutions, both are in the closed interval ![[0,1] [0,1]](http://s.wordpress.com/latex.php?latex=%5B0%2C1%5D&bg=ffffff&fg=000000&s=0) . Find the maximum and minimum values of the expression
. Find the maximum and minimum values of the expression
TOWARD MATHEMATICAL OLYMPIAD
T9/411. Let  and
and 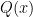 be two polynomials with real coefficients, each has at least one real solution, so that
be two polynomials with real coefficients, each has at least one real solution, so that
Prove that  .
.
T10/411. Let 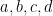 be positive numbers such that
be positive numbers such that  and
and 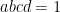 . Find smallest constant k such that the following inequality holds
. Find smallest constant k such that the following inequality holds
T11/411. Find all continuous functions 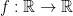 satisfying
satisfying
Where ![[t] [t]](http://s.wordpress.com/latex.php?latex=%5Bt%5D&bg=ffffff&fg=000000&s=0) is the largest integer not exceed
is the largest integer not exceed  and
and ![\{t\}=t-[t] \{t\}=t-[t]](http://s.wordpress.com/latex.php?latex=%5C%7Bt%5C%7D%3Dt-%5Bt%5D&bg=ffffff&fg=000000&s=0) .
.
T12/411. Let  be a triangle,
be a triangle, 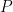 ia an arbitrary point inside the triangle. Let
ia an arbitrary point inside the triangle. Let  be respectively the distances from
be respectively the distances from 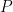 to
to 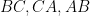 .
.  are the circumradii of triangle
are the circumradii of triangle  respectively. Prove that
respectively. Prove that
No comments:
Post a Comment